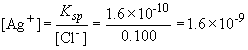
For CrO42-, Ksp = [Ag+]2
[CrO42-]
Chemistry Homework Answer Key #7
Chapter 11 - Solubility Equilibria
49. a) The reaction is, AgOH (s) « Ag+ (aq) + OH- (aq)
So, Ksp = [Ag+] [OH-].Neglecting the hydroxide ion present due to the autoionization of water gives the following equilibrium table:
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
Ksp = x2 = 1.5 ´ 10-8x = 1.2 ´ 10-4
Since x was defined as the equilibrium concentration of Ag+, this value is equivalent to the molar solubility of AgOH.
b) When AgOH is dissolved in a solution that is buffered to a pH of 7.00, it is assumed that [OH-] concentration is fixed at 1.00 ´ 10-7 M and does not change upon the addition of the AgOH (that's what buffers do). So, the equilibrium table looks like:
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
Ksp = x (1.0 ´ 10-7) = 1.5 ´ 10-8x = 0.15 M
So, in this case, the silver concentration is about three orders of magnitude greater than in pure water.
51. The solubility of both AgOH and Ca3(PO4)2
will increase at lower pH since both of the anions are basic. The case
of AgOH is clear; as hydronium increases, hydroxide must decrease. Since
Ksp
= [Ag+] [OH-], anything that decreases the concentration
of hydroxide allows [Ag+] to increase.
The phosphate ion, PO43-, is a weak base. It reacts with hydronium in the following manner:H3O+ + PO43- « HPO42- + H2O
By LeChâtelier's Principle, as H3O+ increases, it forces the equilibrium to the right, thereby decreasing the concentration of PO43-. Since Ksp = [Ca2+]3 [PO43-]2, a decrease in phosphate concentration will result in an increase in the equilibrium concentration of Ca2+.
In contrast, the solubility of PbI2 will not be affected by pH since neither ion acts as an acid or a base (I- is the conjugate base of a strong acid, that is, it has not tendency to hydrolyze).
95. To determine which species precipitates first, we solve for
the equilibrium concentration of silver based on the concentrations of
the anions present:
For Cl-, Ksp = [Ag+] [Cl-]
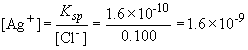
For CrO42-, Ksp = [Ag+]2 [CrO42-]
So, despite the fact that it has a larger Ksp, AgCl will precipitate out of solution first. This is a nice illustration of the fact that simple comparisons of Ksp values for different compounds do not provide reliable estimates of relative solubilities.
When silver chromate begins to precipitate, [Ag+] = 2.8 ´ 10-5. At the concentration the remaining chloride concentration is:
This is 5.7 ´ 10-3 % of the initial chloride concentration; 99.994% of the chloride has been removed when chromate begins to precipitate.
Additional Problem:
In class we derived the relationship between solubility and pH for a simple 1:1 ionic compound, the anion of which is weakly basic. For CuN3, the equation is:
where Ka is the acid ionization constant for the conjugate acid of the anion. In this problem, the constants are: Ksp = 4.9 ´ 10-9 and Ka = 2.2 ´ 10-5. Using the constants in the above equation and solving for [Cu+] at various [H3O+] yields the following plot:
Chapter 12 - Electrochemistry
1 a) 2 VO2+ (aq) + SO2 (g) ® 2 VO2+ (aq) + SO42- (aq)
b) 2 H2O (l) + SO2 (g) + Br2 (l) ® 2 Br- (aq) + SO42- (aq) + 4 H+ (aq)3. a) 2 Cr(OH)3 (s) + 3 Br2 (aq) + 10 OH- (aq) ® 2 CrO42- + 6 Br- + 8 H2O (l)c) Cr2O72- (aq) + 3 Np4+ (aq) + 2 H+ (aq) ® 3 NpO22+ (aq) + 2 Cr3+ (aq) + H2O (l)
d) 2 MnO4- (aq) + 5 HCOOH (aq) + 6 H+ (aq) ® 5 CO2 (g) + 2 Mn2+ (aq) + 8 H2O (l)
e) 2 Au (s) + 8 Cl- (aq) + 3 Hg2HPO4 (s) + 3 H+ (aq) ® 2 AuCl4- (aq) + 6 Hg (l) + 3 H2PO4- (aq)
b) ZrO(OH)2 (s) + 2 SO32- (aq) ® Zr(s) + 2 SO42- (aq) + H2O (l)c) 7 HPbO2- (aq) + 2 Re (s) ® 7 Pb (s) + 2 ReO4- (aq) + H2O (l) + 5 OH- (aq)
d) 4 HXeO4- (aq) + 8 OH- (aq) ® Xe (g) + 3 XeO64- + 6 H2O (l)
e) N2H4 (aq) + 2 CO32- (aq) ® 2 CO (g) + N2 (g) + 4 OH- (aq)
7. 3 HNO2 (aq) ®
2 NO (g) + NO3- (aq) + H+
(aq) + H2O (l)
10. The galvanic cell given is: Ni(s) | Ni2+ (aq) || HCl (aq) | H2 (g) | Pt (s)
Using this notation, the anode is always on the left-hand side and the cathode is on the right. Electrons will flow from the nickel anode to the platinum cathode; anions (Cl-) will flow from the cathodic cell to the anodic cell via the salt bridge.
11. At the cathode in this cell, the half-reaction is:
Sn4+ (aq) + 4 e- ® Sn (s)
The amount of metallic tin that can be deposited at the cathode is:13. At the anode, the half-reaction is: Zn (s) ® Zn2+ (aq) + 2e-
At the cathode, the half-reaction is: Cl2 (g) + 2 e- ® 2 Cl- (aq)Adding these reactions together yields: Zn (s) + Cl2 (g) ® Zn2+ (aq) + 2 Cl- (aq)
b) The total charge passed is:

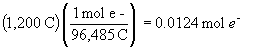
c) the change in mass of the zinc anode is:
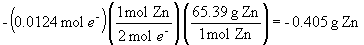
d) the volume of chlorine gas consumed (it is being reduced to chloride) is:
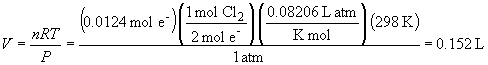
17. The overall reaction is: KCl (l) ®
K (l) + 1/2 Cl2 (g)
The oxidation (at the anode) half-reaction is: Cl-® 1/2 Cl2 (g) + e-The reduction (at the cathode) half-reaction is: K+ + e-® K (l)
The mass of products is given by: