ß is the ratio of the pressure of the gas to the pressure
of the magnetic field of a plasma.
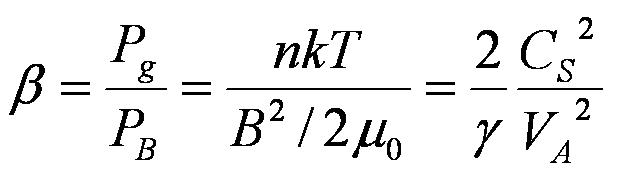
Pg = gas pressure
PB = magnetic field pressure
n = particle density
k = Boltzmann's constant
T = temperature
B = magnetic field strength
μ0 = permeability of free space
CS = sound speed in plasma
VA = Alfvén speed
A classic cartoon to describe plasma in a simple way is the
rubber-band-in-gelatin model. Remember that plasma is an ionized
gas of positive ions and negative electrons. It is overall electrically
neutral and it has an associated magnetic field. We can think of the ionized
gas of the plasma as a blob of gelatin. While the gelatin was still
liquid we laid some rubber bands down in it so that when the
gelatin congealed the rubber bands were suspended in it. We can
think of the rubber bands as the magnetic field of the plasma.

When ß>1, the gas pressure dominates the plasma. In
other words the gelatin dominates the movement of the rubber
bands. So, if we jiggle the gelatin, the rubber bands will move.
When ß<1, the magnetic pressure dominates the plasma.
In this case, if we jiggle a rubber band, the gelatin will move.
When ß~1, neither the gas pressure nor the magnetic pressure
dominates the plasma. This condition allows for resonance of
waves in the plasma. A magnetic wave in this plasma can, in a
sense, choose to become an acoustic wave or vice versa.
Next: How can a magnetic
wave transform into an acoustic wave?
How are aurorae created?
Can we hear the aurorae?
Wave transformation on
the Sun
What is ß?
How can a magnetic wave
transform into an acoustic wave?
Does ß=1 anywhere in Earth’s
atmosphere?
Continuing research
Bibliography
